式の計算の練習問題1
オンライン塾始めました。
6ヶ月で自分で勉強できるようにサポートします。
1751 Views
2019年3月16日式の計算中学2年生
問1
次の計算をしましょう。
(1)$4(2x-5)$
(2)$\displaystyle \frac{2a-1}{3}+\displaystyle \frac{7a+3}{6}$
(3)$(1.2x²+0.3x)-(0.4x²-0.7x)$
(4)$(-3a²+6ab²-9a)÷(-3a)$
(1)
$=8x-20$
(2)
$=\displaystyle \frac{4a-2+7a+3}{6}$
$=\displaystyle \frac{11a+1}{6}$
(3)
$=1.2x²+0.3x-0.4x²+0.7x$
$=0.8x²+x$
(4)
$=a-2b²+3$
問2
次の式の値を求めましょう。
(1)$\displaystyle \frac{a+2b}{4}-\displaystyle \frac{a+3b}{6}$$(a=-4,b=6)$
(2)$4ab÷(-6b)²×9bc$$(a=-\displaystyle \frac{1}{2},b=4,c=-1)$
(1)
$=\displaystyle \frac{3a+6b}{12}-\displaystyle \frac{2a+6b}{12}$
$=\displaystyle \frac{3a+6b-2a-6b}{12}$
$=\displaystyle \frac{a}{12}$
$=\displaystyle \frac{-4}{12}$
$=-\displaystyle \frac{1}{3}$
(2)
$=4ab÷36b²×9bc$
$=\displaystyle \frac{4ab×9bc}{36b²}$
$=ac$
$=-\displaystyle \frac{1}{2}×(-1)$
$=\displaystyle \frac{1}{2}$
問3
連続した3つの整数の和は3の倍数になります。このことを説明しましょう。
ポイントは
連続した3つの整数は1つを文字で置くと表せる。
連続した3つの整数は整数$n$を使って以下のように表せる。
$n$,$n+1$,$n+2$
これらの和は
$n+n+1+n+2$
$=3n+3$
$=3(n+1)$
$n$は整数なので、連続した3つの整数の和は3の倍数になる。

問4
半径が$rcm$、高さが$hcm$、体積が$Vcm³$の円錐があります。
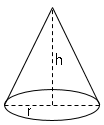

(1)体積を求める式を書きましょう。
(2)$h$について解きましょう。
(3)底面の半径が$3cm$,体積が$36πcm³$の円錐の高さを求めましょう。
(1)
円錐の体積$=$底面積$×$高さ$×\displaystyle \frac{1}{3}$
$V=r×r×π×h×\displaystyle \frac{1}{3}$
$V=\displaystyle \frac{πhr²}{3}$
(2)
$V=\displaystyle \frac{πhr²}{3}$なので
$3V=πhr²$
$h=\displaystyle \frac{3V}{πr²}$
(3)
$h=\displaystyle \frac{3V}{πr²}$なので、
$h=\displaystyle \frac{3×36π}{π×3²}$
$h=12$
円錐の高さは$12cm$
問5
$5$で割ると$2$余る整数と$5$で割ると$3$余る整数の和は$5$の倍数になることを文字を使って説明しましょう。

整数を$n,m$、商を$a,b$(ともに整数)とすると、
$n=5×a+2$、$m=5×b+3$となる。
これらの和は
$5×a+2+5×b+3$
$=5a+5b+5$
$=5(a+b+1)$
$a,b$は整数なので、整数の和は$5$の倍数になる。
動画で理解しよう!ここまでの内容だよ

不明点があればコメントよりどうぞ。











ディスカッション
コメント一覧
まだ、コメントがありません